Use your addition and subtraction skills, combined with some strategic thinking, to beat your partner at this game.
Ages 5 – 11
The player who stops their opponent from being able to go wins the game.
How to play:
- Start by drawing a number line from 0 to 20 like this:
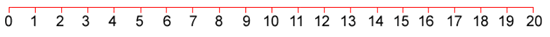
(You can find some of these number lines here.)
- The first player chooses a number on the line and crosses it out. The same player then chooses a second number and crosses that out too. Finally, he or she circles the sum or difference of the two numbers and writes down the calculation.
For example, the first player’s go could look like this:
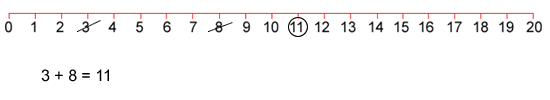
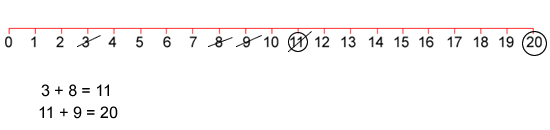
- The second player must start by crossing off the number that player 1 has just circled. He or she then chooses another number to cross out and then circles a third number which is the sum or difference of the two crossed-off numbers. Player 2 also writes down their calculation.
For example, once the second player has had a turn, the game could look like this:
- Play continues in this way with each player starting with the number that has just been circled.
- Once a number has been used in a calculation, it cannot be used again.
- The game ends when one player cannot make a calculation. The other player is the winner.
It’s your turn!
Try playing the game against someone else a few times to get a feel for it.
Do you have any good ways of winning?
Now it’s time to work together with a partner, rather than against them.
Try to create a string of calculations that uses as many numbers as possible on the 0-20 number line.
Is it possible to create a string of number sentences that uses all the numbers on the 0-20 number line? Why or why not? How would you convince a mathematician?
Once you’ve had a good think about it, you may like to look at this proof that has been scrambled up.
Can you rearrange it into its original order?
If you would prefer to work away from a screen, you could print off, cut up and rearrange the statements on this sheet (it includes three copies of each statement).
What about the 1-20 number line? Is it possible to create a string of number sentences that uses all the numbers on the 1-20 number line? Why or why not? How would you convince a mathematician?
Again, once you have thought about it, you may like to look at this proof that has been scrambled up.
Can you rearrange it into its original order?
If you would prefer to work away from a screen, you could print off, cut up and rearrange the statements on this sheet (it includes two copies of each statement).